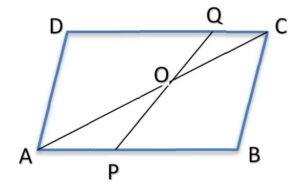
Q) ABCD is a parallelogram. Point P divides AB in the ratio 2:3 and point Q divides DC in the ratio 4:1. Prove that OC is half of OA.
Ans:
Given that ABCD is a parallelogram. Therefore, AB ǁ CD and BC ǁ AD
Since, Point P divides AB in the ratio 2:3
Therefore, if AB = a, then AP = ![]() a and BP =
a and BP = ![]() a
a
Since, Point Q divides CD in the ratio 4:1
Therefore, since CD = AB = a, then DQ = ![]() a and QC =
a and QC = ![]() a
a
Let’s look at Δ AOP and Δ QOC,
∠ AOP = ∠ QOC (vertically opposite angles)
∠ OAP = ∠ QCO (interior angles)
Therefore, Δ AOP ![]() Δ QOC
Δ QOC
Hence, ![]() =
= ![]()
![]() ,
, ![]() =
= ![]()
![]() =
= ![]()
OC = ![]() OA
OA
Therefore, it is proved that OC is half of OA.
