Q. Computer-based learning (CBL) refers to any teaching methodology that makes use of computers for information transmission. At an elementary school level, computer applications can be used to display multimedia lesson plans. A survey was done on 1000 elementary and secondary schools of Assam and they were classified by the number of computers they had.

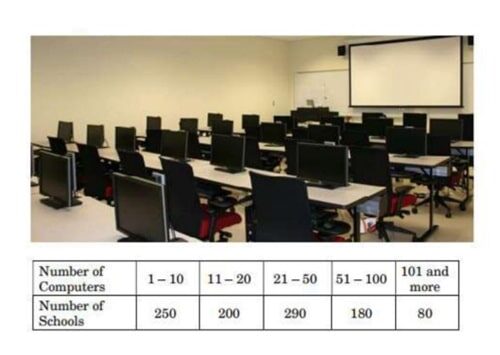
One school is chosen at random. Then:
(i) Find the probability that the school chosen at random has more than 100 computers.
(ii) (a) Find the probability that the school chosen at random has 50 or fewer computers.
OR
(ii) (b) Find the probability that the school chosen at random has no more than 20 computers.
(iii) Find the probability that the school chosen at random has 10 or less than 10 computers.

Ans:

VIDEO SOLUTION

 

STEP BY STEP SOLUTION

 

(i) Probability of choosing a school with more than 100 computers:

=

= = 0.08

Therefore, Probability of choosing a school with more than 100 computers = 0.08

(ii) (a) Probability to choose a school with 50 or less computers:

=

=

= = 0.74

Therefore, Probability to choose a school with 50 or less computers = 0.74

OR

(ii) (b) Probability to choose a school with no more than 20 computers:

=

=

= = 0.45

Therefore, Probability to choose a school with no more than 20 computers = 0.45

(iii) Probability to choose a school with 10 or less than 10 computers:

=

= = 0.25

Therefore, Probability to choose a school with no more than 20 computers = 0.25