Q) In the given figure, tangents PQ and PR are drawn to a circle such that ∠RPQ = 30⁰. A chord RS is drawn parallel to tangent PQ. Find the ∠RQS.

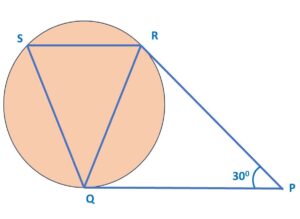
Ans: In △PRQ, PQ and PR are tangents from an external point P to circle.

∴ PR = PQ

Since the angles opposites to equal sides are equal

∴ ∠PRQ = ∠PQR

Now, by Angle sum property, in △PRQ, ∠PRQ + ∠PQR + ∠RPQ = 180⁰

∵ ∠RPQ = 30⁰

∴ ∠PRQ + ∠PRQ + 30⁰ = 180⁰

∴ 2 ∠PRQ  + 30⁰ = 180⁰

∴  ∠PRQ  = 75⁰

Therefore, ∠PRQ= ∠PQR = 75⁰

Since PQ ∥ SR, and RQ cuts these 2 lines:

∴ ∠PQR = ∠SRQ = 75⁰   (Alternate angles)

Since PQ is tangent at Q and QR is chord at Q.

∴ ∠RSQ = ∠PQR = 75⁰  (∠RSQ in alternate segment of circle]

Now, In △SRQ,

∵ ∠RSQ + ∠SRQ + ∠SQR = 180⁰   (Angle sum property of a triangle)

∴ 75⁰  + 75⁰  + ∠SQR = 180⁰

∴  ∠SQR = 180⁰ – 150⁰ 

∴  ∠SQR = 30⁰